Shocks

Shock Fronts

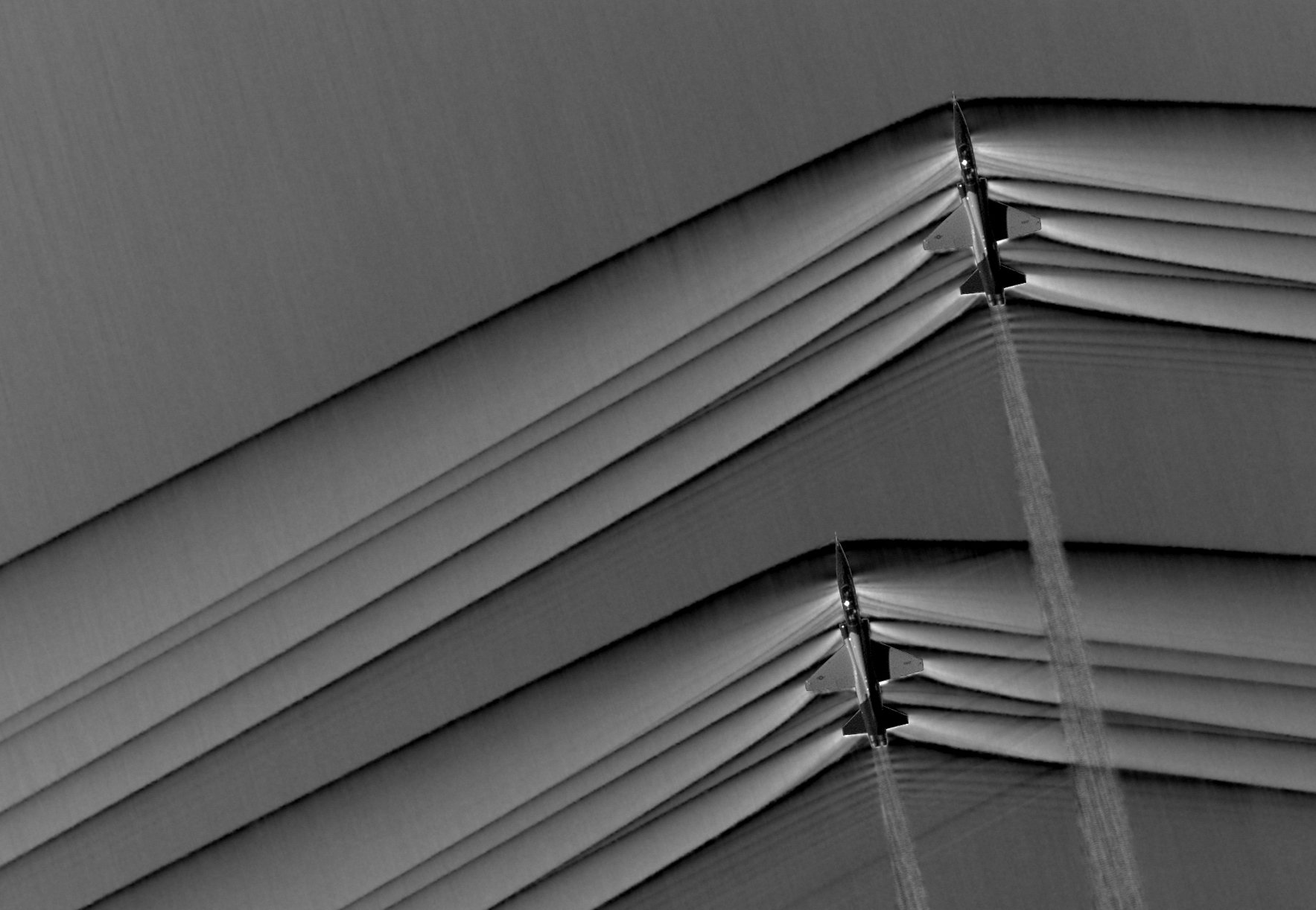
The photo at the top of the page shows examples of the shock fronts that accompany a jet flying at supersonic speed through Earth’s atmosphere. Sound waves coming from the jet travel more slowly through the atmosphere than the jet does. Therefore, those sound waves don’t disturb the air ahead of the jet. Instead of arriving gradually, in a series of pressure waves, the pressure disturbance moving with the jet arrives at all once, in the form of a shock front.

Thunder claps are naturally occurring shock fronts resulting from lightning bolts. The bolt releases electrical energy that strongly heats neighboring air, which rapidly expands. A pressure impulse results and moves faster than the speed of sound into air farther from the bolt.

Exploding stars produce similar shock fronts in a galaxy’s atmosphere. Matter flying away from an exploding star can drive a pressure impulse faster than the speed of sound into the surrounding gas. The resulting shock front then expands outward through the galactic atmosphere.

A Wall of Gas

Propelling gas at supersonic speed into a motionless wall also results in a shock front. The wall stops the incoming gas. Sound waves produced as the incoming gas decelerates cannot propagate upstream faster than the supersonic flow carries them toward the wall. The result is a pileup of motionless gas between the wall and the supersonic flow, with a shock front at the boundary between the motionless gas and the incoming flow.

The shock front propagates away from the the wall at a speed that depends on how much the shock compresses the incoming gas. From the viewpoint of an observer moving along with the shock front, like the pilot of a supersonic jet, the configuration of the front appears steady, with outgoing flow rates of gas mass and energy equal to the incoming flow rates. But from the point of view of an observer who is stationary with respect to the pre-shock gas, like a person on Earth watching a supersonic jet pass overhead, the shock front strikes like a sudden wall of gas pressure, arriving at supersonic speed.

Thermalization

Interactions among gas particles within the shock front convert incoming kinetic energy into thermal energy. As the gas flow ramming into a motionless wall sharply decelerates, the random speeds of gas particles relative to each other sharply increase. A shock front therefore boosts the specific entropy of the incoming gas.

An incoming flow of density ${\rho_{\rm pre}}$ and a speed $\Delta v$ relative to the wall has a kinetic energy per per unit mass of $(\Delta v)^2 / 2$. If the incoming thermal energy is negligible compared to the incoming kinetic energy, then the shock front simply scatters the incoming particles so that their velocities go from fully aligned to isotropic. The gas still has the same total energy per unit mass but is now stationary, so the equation $$\frac {kT_{\rm post}} {\gamma - 1} = \frac {\mu m_p (\Delta v)^2} {2}$$determines the postshock temperature $T_{\rm post}$ of gas with postshock pressure $P_{\rm post}$ and thermal energy density $P_{\rm post} / (\gamma - 1)$.

Deceleration

An observer moving along with a shock front sees gas arriving at an incoming speed $v_{\rm pre}$ and decelerating to a slower speed $v_{\rm post}$. In a steady state, the equation $$\rho_{\rm pre} v_{\rm pre} = \rho_{\rm post} v_{\rm post}$$describes the steady flow of gas per unit area through the front and relates the postshock gas density $\rho_{\rm post}$ to the preshock gas density $\rho_{\rm pre}$.

The difference between preshock gas pressure $P_{\rm pre}$ and postshock gas pressure $P_{\rm post}$ causes the momentum of that gas flow to change. Pressure corresponds to a force per unit area acting upon the momentum flow per unit area, which is $\rho v^2$. The equation $$P_{\rm post} - P_{\rm pre} = \rho_{\rm pre} v_{\rm pre}^2 - \rho_{\rm post} v_{\rm post}^2$$therefore describes the magnitude of the change in momentum per unit area flowing across the shock front.

Compression

Combining the effects of thermalization and deceleration determines the compression ratio $${\cal R} = \frac {\rho_{\rm post}} {\rho_{\rm pre}} = \frac {v_{\rm pre}} {v_{\rm post}}$$of the shock front. If the preshock gas pressure and thermal energy density are negligible, we then get $$P_{\rm post} = \left( \frac {1} {{\cal R} - 1} \right) \rho_{\rm post} (\Delta v)^2$$from deceleration and $$P_{\rm post} = \left( \frac {\gamma - 1} {2} \right) \rho_{\rm post} (\Delta v)^2$$from thermalization, with $\Delta v = v_{\rm pre} - v_{\rm post}$.

Equating those expressions and solving for ${\cal R}$ yields $${\cal R} = \frac {\gamma + 1} {\gamma - 1}$$which reduces to ${\cal R} = 4$ for an ideal gas with $\gamma = 5/3$. A strong shock therefore increases the density of that gas by a factor of 4 and lowers its flow speed relative to the shock front to a quarter of the incoming flow speed.

A strong shock in Earth’s atmosphere produces more compression because our atmosphere consists of gas with $\gamma \approx 7/5$. In that case, the compression ratio of a strong shock is ${\cal R} \approx 6$.

Energy Conservation

A shock’s compression ratio is smaller if the incoming thermal energy is comparable to the incoming kinetic energy. In that case, the energy conservation relation $$\Delta \left( \frac {v^2} {2} + \frac {1} {\gamma - 1} \frac {P} {\rho} \right) = - \Delta \left( \frac {P} {\rho} \right)$$determines the postshock temperature. The quantity on the left is the net change in total energy per particle (kinetic plus thermal) across the shock front. The quantity on the right accounts for both the thermal energy that compression adds to the shocked gas and the kinetic energy that becomes thermalized as the shocked gas decelerates.

The energy conservation relation gives $$P_{\rm post} = {\cal R} P_{\rm pre} + \frac {\gamma - 1} {2 \gamma} \left( {\cal R} - \frac {1} {\cal R} \right) \rho_{\rm pre} v_{\rm pre}^2$$for the postshock pressure. Equating that result to the relation $$P_{\rm post} = P_{\rm pre} + \rho_{\rm pre} v_{\rm pre}^2 \left(1 - \frac {1} {\cal R} \right)$$describing deceleration then leads to $${\cal R} = \frac {\gamma + 1} {\gamma - 1} \left( 1 + \frac {2 \gamma} {\gamma -1} \frac {P_{\rm pre}} {\rho_{\rm pre} v_{\rm pre}^2} \right)^{-1}$$for the compression ratio. The form of this equation shows that increasing $P_{\rm pre}$ relative to $\rho_{\rm pre} v_{\rm pre}^2$ reduces a shock’s compression ratio.

Mach Number

The compression ratio of a shock front is typically expressed in terms of a Mach number $${\cal M} = \frac {v_{\rm pre}} {c_{\rm s,pre}}$$that relates the incoming flow speed $v_{\rm pre}$ to the sound speed ${c_{\rm s,pre}} = ( \gamma P_{\rm pre} / \rho_{\rm pre} )^{1/2}$in the preshock gas. When written in terms of Mach number, the shock’s compression ratio becomes $${\cal R} = \frac {\gamma + 1} {\gamma - 1} \left( 1 + \frac {2} {\gamma -1} {\cal M}^{-2} \right)^{-1}$$and the compression ratio converges toward $${\cal R} = \frac {\gamma + 1} {\gamma - 1}$$as ${\cal M}$ becomes large.