Stability

Perturbations

We consider an atmosphere stable if small disturbances within it do not grow to become large disturbances. For example, we can represent a pressure disturbance mathematically as a difference $$\delta P = P - {\bar P}$$between the local pressure $P$ and the mean pressure ${\bar P}$ of gas at the same altitude. A small pressure perturbation $\delta P \ll P$ will cause a small rearrangement of the atmospheric gas. In a stable atmosphere, that small rearrangement does not produce pressure perturbations that are larger than the original one.

Sound Waves

The disturbances known as sound waves result from periodic compressions of atmospheric gas. Compression in the $x$ direction can be expressed in terms of changes in position of atmospheric gas that depend on the $x$ coordinate of that gas. In a sound wave, the changes in position depend on both location $x$ and time $t$, and compression happens wherever gas flows in two neighboring regions are moving toward each other.

Small position changes $\delta x$ that depend on location $x$ can produce small density perturbations $$\delta \rho = -{\bar \rho} \frac {\partial} {\partial x} \delta x$$in an atmosphere with a mean gas density ${\bar \rho}$. They can also produce small pressure perturbations $$\delta P = \: \gamma {\bar P} \frac {\delta \rho} {\bar{\rho}} = \: - \gamma {\bar P} \frac {\partial} {\partial x} \delta x$$if gas near the mean atmospheric pressure ${\bar P}$ obeys the equation of state $P \propto \rho^\gamma$.

The pressure perturbations cause accelerations that change the atmospheric velocity $v_x$ in the $x$ direction according to $$\frac {d v_x} {d t} = - \frac {1} {\bar \rho} \frac {\partial} {\partial x} \delta P = \frac {\gamma \bar P} {\bar \rho} \frac {\partial^2} {\partial x^2} \delta x$$if gravity can be neglected. In a motionless atmosphere, we then obtain $$\frac {\partial^2} {\partial t^2} \delta x = \frac {\gamma \bar P} {\bar \rho} \frac {\partial^2} {\partial x^2} \delta x$$by substituting $\partial^2 (\delta x) / \partial t^2$ for $dv_x/dt$.

Experienced physics students will recognize that the resulting equation for $\delta x$ has wavelike solutions. Substituting either $$\delta x \propto \sin (x - c_{\rm s} t)$$or $$\delta x \propto \sin (x + c_{\rm s} t)$$solves the equation, as long as $c_{\rm s}^2 = \gamma {\bar P} / {\bar \rho}$. These wavelike disturbances move at a speed $$c_{\rm s} = \sqrt { \frac {\gamma {\bar P}} {\bar \rho}}$$in either the $+x$ or $-x$ direction. In other words, $c_{\rm s}$ is the speed of sound waves in an atmosphere with $P = K \rho^\gamma$ and constant $K$.

Importantly, sound waves in an atmosphere with constant $K$ are stable, because there is not an energy source making them grow with time. The compressions and rarefactions of gas are periodically exchanging the same total amount of kinetic plus thermal energy. More complete analyses of sound waves show that they gradually decay as they dissipate their energy into heat.

Internal Gravity Waves

A different kind of atmospheric disturbance arises from wavelike perturbations that lift and lower gas in neighboring regions. Lifting atmospheric gas by a small amount $\delta r$ changes the quantity $K$ at radius $r$ by an amount $$\delta K = - \frac {d {\bar K}} {dr} \delta r$$in an atmosphere with a mean entropy ${\bar K}(r)$ at altitude $r$. Differences in the amount of lifting from place to place can therefore produce differences in the buoyancy of the gas there.

Sound waves propagating more rapidly than the lifting motions will tend to equalize the gas pressures at a given altitude. In that case, small entropy perturbations lead to small density perturbations $$\delta \rho = - \frac {\bar{\rho}(r)} {\gamma {\bar{K}(r)}} \delta K = \frac {\alpha} {\gamma} \frac {\bar{\rho}(r)} {r} \delta r$$in which $$\alpha = \frac {r} {\bar{K}} \frac {d {\bar K}} {dr}$$ represents how ${\bar K}$ changes with radius.

Because of buoyancy, these density perturbations then accelerate either upward or downward according to $$\frac {\partial^2} {\partial t^2} \delta r = - g \frac {\delta \rho} {\bar \rho} = - \omega_g^2 \, \delta r$$in which the quantity $$ \omega_g^2 = \frac {\alpha g} {\gamma r}$$has a positive value only if specific entropy rises with radius, giving $\alpha > 0$. If that condition holds, then density perturbations with $\delta r \propto \sin \omega_g t$ are solutions to the equation. They are known as internal gravity waves, and they oscillate with a frequency $\omega_g$ in this simplified example.

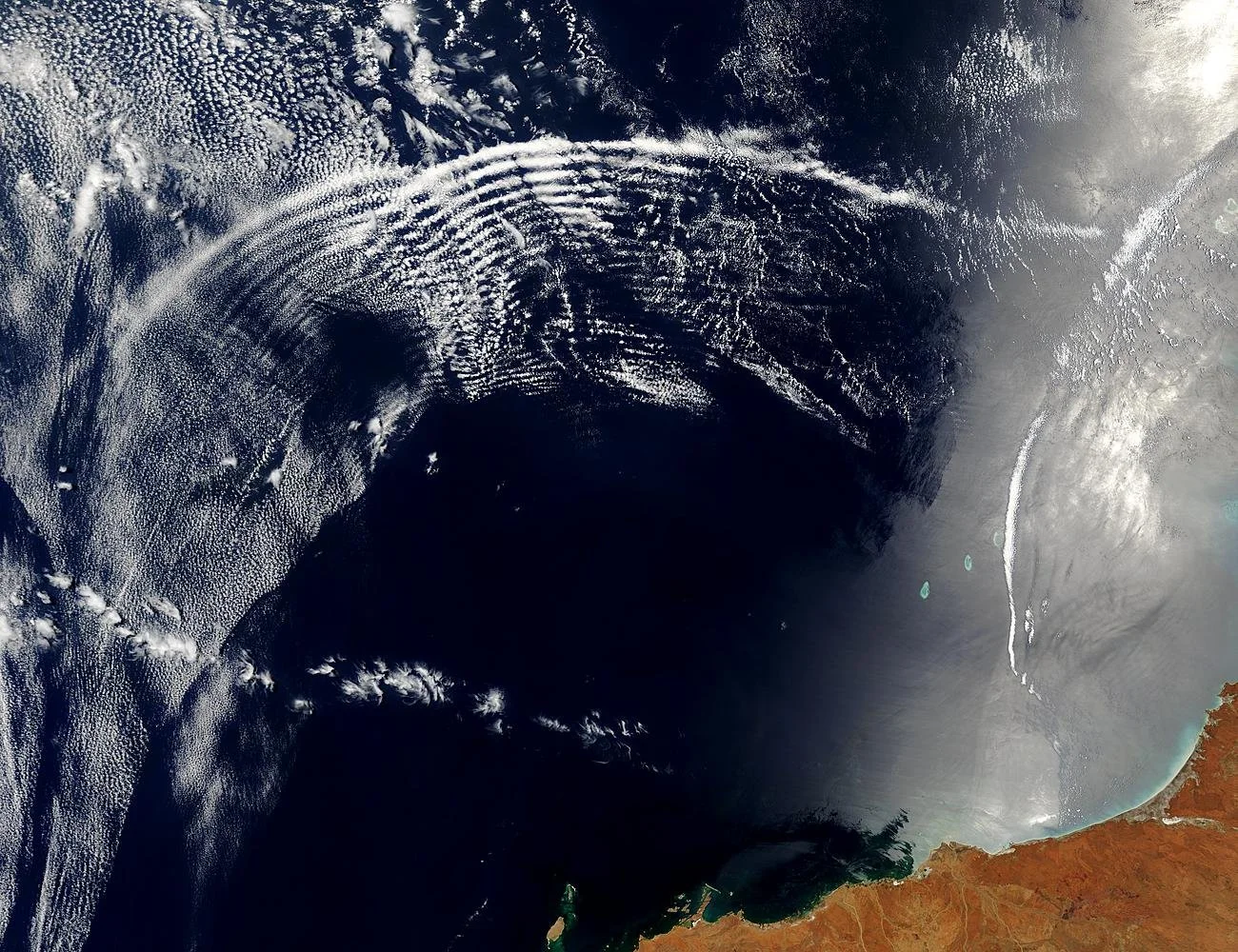
The photo at the top of this page shows some internal gravity waves in Earth’s atmosphere, where rippling clouds are tracing the up-and-down bobbing of atmospheric gas. This phenomenon is closely related to the waves that form on a body of water. Both kinds of waves bob up and down for the same reason. Material gets pushed up above the altitude at which it is in equilibrium, making it denser than average for its altitude. Gravity then pulls it back down. Those impulses result in oscillatory motion in which the total amount of gravitational potential energy plus kinetic energy remains constant.

Instability

Like sound waves, internal gravity waves tend to be stable if there is no source of energy pumping their motion and increasing the total amount of wave energy. But the disturbances that produce internal gravity waves lead to oscillatory motion only in atmospheres in which specific entropy rises with radius.

Those same disturbances are unstable in an atmosphere in which specific entropy declines with increasing altitude ($d {\bar K} / dr < 0$). In that case, solutions of the equation for the time dependence of $\delta r$ have the form $\delta r \propto e^{|\omega_g| t}$, meaning that the amplitudes of initially small perturbations grow exponentially with time. The timescale for exponential growth is $$\left( \frac {\gamma r} {|\alpha| g} \right)^{1/2} = \left( \frac {\gamma} {2 |\alpha|} \right)^{1/2} t_{\rm ff}$$in which $t_{\rm ff} = ( 2r / g)^{1/2}$ is a free-fall timescale based on the local gravitational acceleration $g$ at radius $r$. (Something starting at rest and accelerating at the rate $g$ for a time $t_{\rm ff}$ travels a distance $r$.)

An atmosphere with low entropy gas resting on high entropy gas is unstable because gradual lifting of a particular region of the atmosphere makes it less dense that its surroundings, and therefore more buoyant. As that gas rises further, its density contrast increases, making it even more buoyant and spurring a runaway rise toward greater altitudes. Gradual lowering of an atmospheric region leads to the opposite outcome—a runaway plunge to lower altitudes.

This mode of instability is known as a Rayleigh-Taylor instability. It is just one of many ways for an atmosphere to be unstable.

Convection

Earth’s atmosphere can become unstable as sunlight heats it from below. Rays of sunshine heat Earth’s surface, which then transfers that heat to the air immediately above it. On a sunny day, the added heat raises the specific entropy of surface air above the specific entropy of air at greater altitudes. It therefore rises away from the surface while lower-entropy air descends around it.

This process of energy transport is called convection. In an atmosphere, it results from the condition $d {\bar{K}} / dr < 0$. The rising of hot air and falling of cool air continues to transport heat energy upward until $d {\bar{K}} / dr = 0$. Much of what happens in Earth’s lowest atmospheric layer, the troposphere, therefore depends on the process of convection.

Active star formation within a galaxy also drives convection, particularly when those stars explode. Supernova explosions strongly heat nearby atmospheric gas, producing high-entropy bubbles that can rise through the galaxy’s atmosphere. Galaxies therefore display phenomena analogous to Earth’s weather, driven by convection and sometimes shaped by Coriolis acceleration stemming from the galaxy’s rotation.